At present there is a rather extensive number of the geophysical facts difficulty explainable from conventional positions. Indeed, geoid in the third approximation has form of cardioidal ellipsoid with the depression at the South pole and the prominence at the North one. Coefficient of asymmetry h » 10^-5 (King-Hele et al. 1959). Scarcely it might be explained accidental concentration of mass in the northern hemisphere, since analogical asymmetry have been found in figures of the other planets (Kozyrev 1950). Obviously, it is caused by some low-governed asymmetrical forces directed along the axis of rotation. It have been found that coefficient of asymmetry is directly connected with velocity of planetary rotation. However in conventional mechanics any asymmetrical forces connected with rotation are absent.

Zonal distribution of the land and sea is also asymmetrical (Shubaev 1958) and describes by cardioid opposite to geodesical one. There are some evidences (Dragunov 1960) that such distribution conserves as whole by averaging over time order to 10⁸ years in spite of the processes of continental drift and movement of the pole.

In the atmosphere there is heat asymmetry of the hemispheres (Khromov et al. 1971) (northern hemisphere is 3° higher than southern one), asymmetry of the intertropic convergence zone and other indications of asymmetry. One can mention law-governed asymmetry of the Earth’s magnetic field noted for a long time (Bauer 1923, Pushkov et al. 1975).

This section is dedicated to evaluation of theoretical distribution of force of causality on the Earth and analysis of its geophysical effects.

Force of causality acting on a unit volume in the rotating Earth, on the base (8) of section by S.M.Korotaev in chapter I can be written as

, (1)

where w is angular velocity, r distance from center of the Earth, j is geocentrical latitude, Dj is the difference of geographical and geocentrical latitudes. Since Dj vanishes, then

. (2)

The force acts over the all points of the Earth parallely Earth’s axis to the north. Conservation of impulse requires the force of reaction to be directed parallel to the axis to the south, so

, (3)

where integration conducts over all the volume of the Earth. By this it is possible to consider the integral reaction applied to the center of the Earth that is equivalent to its uniform distribution over sphere of radius r.

Then we have a solution:

. (4)

Resulting force at the latitude j is

. (5)

For geophysical interpretation of the obtained expression, the following is essential. As mentioned above considered force has opposite sign for causes and consequences. Having not a possibility to apply strict approach developed in (Korotaev 1992), it can define cause and consequence objectively by direction of free energy flow: that is directed always from cause to consequence. Solid body of the Earth loses heat to environmental space. Therefore it might be considered as “the region of causes”. On considering the system solid Earth — atmosphere, by analogous way it is easy to see that atmosphere is in “the region of consequences”.

In (5) the sign is selected for causes therefore (5) should be taken with its sign for the solid Earth and with opposite one for the atmosphere.

Calculation of the force by (5) was found to be in good agreement with the direct measurements (Kozyrev 1958, 1963, 1971). The latter, in particular, has shown an existence of the critical parallel 73° 05ў ± 05ў where, force of causality changes its sign. (5) gives theoretical estimation ~70° 20ў , that is good coincidence, taking into account the assumed approximations Dj = 0, r = r(r), i.e. g =const under r =const.

Dependence (j) is shown in Fig.1 (positive direction is parallel to the Earth’s axis to the north).

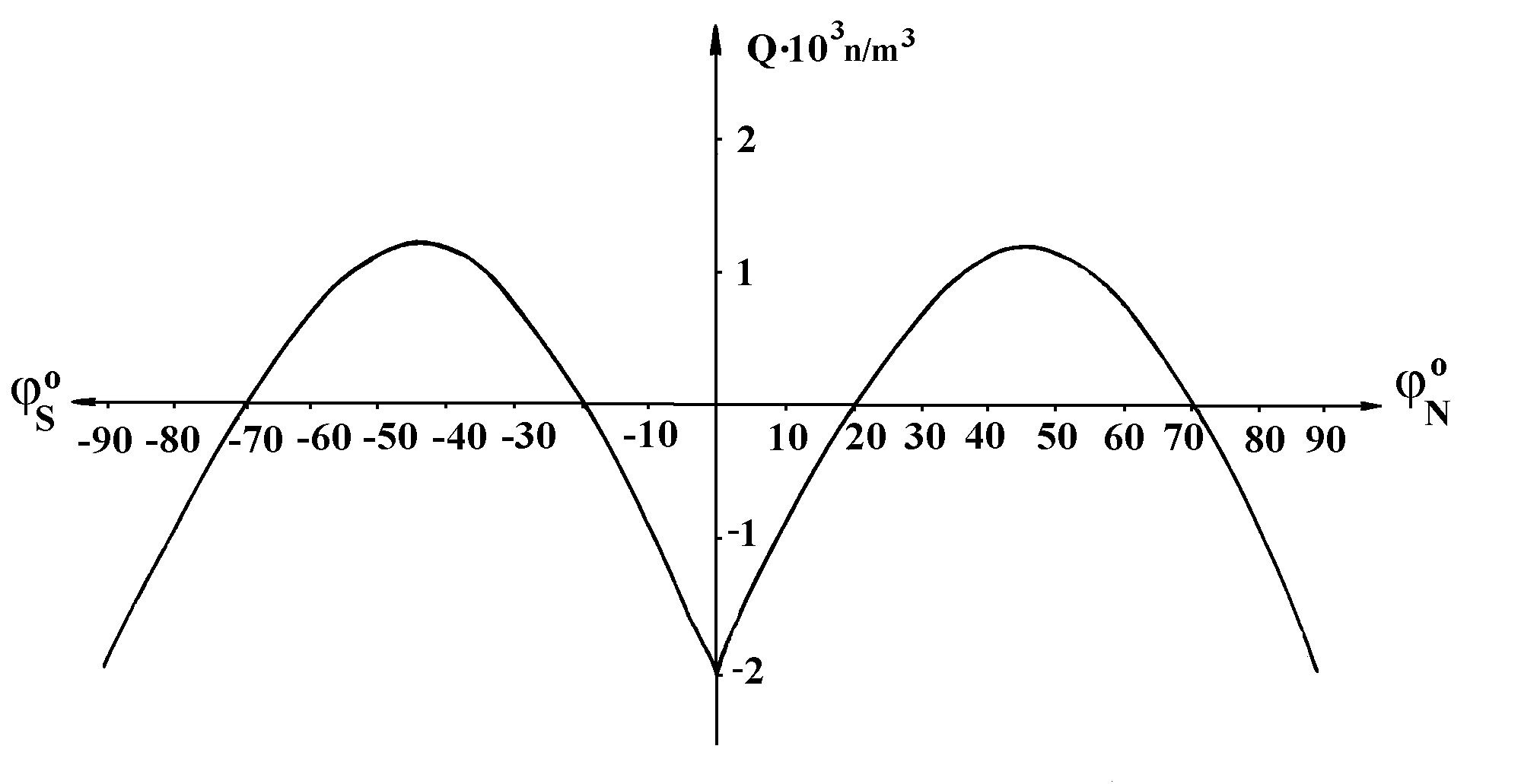
Fig.1. Zonal distribution of force of causality (for r = 1 kg/m³ ).

Since vertical and horizontal forces in geophysics play essentially different roles, they can be considered separately.

Vertical component Q_R is:

, (6)

horizontal one Q_j is

. (7)

In the geological time scale the result of those forces can be noticed through their divergencies:

, (8)

, (9)

(the sign plus is for the northern hemisphere, the sign minus — for the southern one).

Extraction at the process of differentiation of mantle matter more light granitoid fraction controlled in the main, by reaction (Magnitsky 1958):

MgSiO_3® MgSiO₄+SiO₂

go quicker by decreasing of pressure, i.e. under condition of vertical stretching (divQ_R>0). Therefore, zones where divQ_R>0 are more favourable for forming of the continental crust than zones where divQ_R<0. Zones with divQ_R<0 are more favourable for the opposite process basification, although the latter has in nature limited significance (concretely genesis of the eastern segment of the Arctic ocean may be explained by such mechanism (King et al. 1964, Demenitskaya et al. 1971)).

Comparison of the curve of distribution of land and sea and the curve divQ_R(j) show (Fig.2) their excellent coincidence. Taking into account their non-trivial look, one can assert that namely field of force of causality, having an effect on the processes of differentiation has had decisive influence on forming of zonal distribution of the crust types.

Formula (8) gives physical explanation, previously morphologically detected evidences of the Earth’s asymmetry relatively equator: the circles of polar antisymmetry (± 71° ), the northern epeirogenetic (+62° ) and the southern talassogenetic (-62° ) circles (Katterfeld 1962).

On the other hand, horizontal component of the force must bring an additional contribution to the process of continental drift. Since force of causality is a permanent factor, one can expect concentration of movable continental masses in the zones of convergence of the horizontal force (divQ_j<0) and deficit in the zones of divergence (divQ_j>0). The curve divQ_j(j) is presented in Fig.2. It is evidently clear, the continental zones qualitatively correspond to the zones of convergence, the oceanic ones correspond to the zones of divergence.

It is of interest to consider rotor of the force :

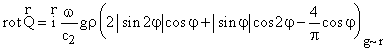(10)

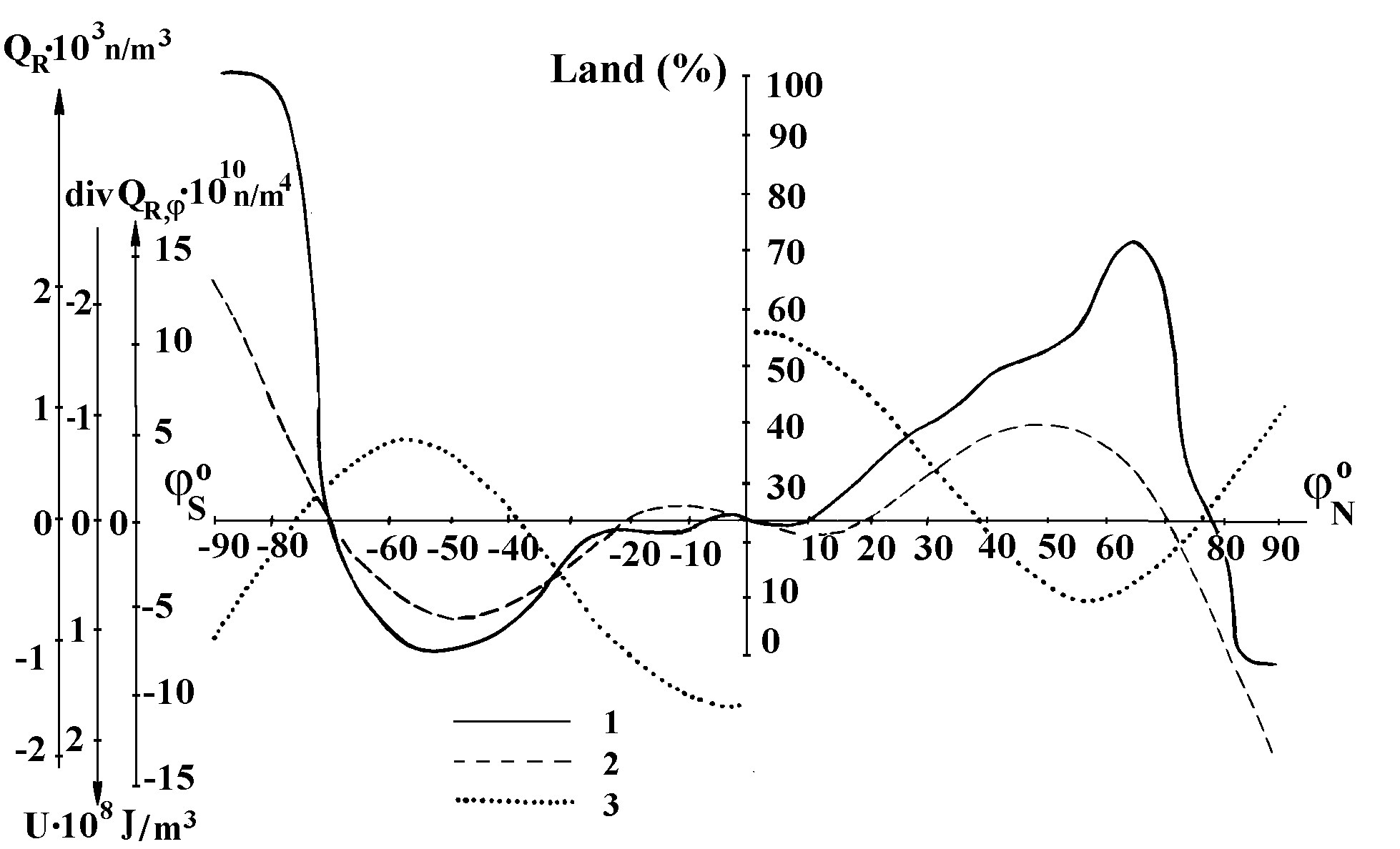
Fig.2. Zonal distribution of the land (1), vertical component (2), divergence of vertical (2) and horizontal (3) components and potential (2) of force of causality (for r = 1 kg/m³).

(positive direction of rot is to the east). Rotor of the force determines intensity of shift deformation and, therefore, extremal values of rot (j) on the Earth must correspond the orogenic belts or zones of the deep faults. Figure 3 shows, that extrema really correspond in particular, to known critical parallels ± 35° and the equator (the formers on the continents are orogenic belts, the latter is expressed as fault zone) (Katterfeld 1962).

Vertical component of force of causality (6) is also responsible for deformation of the balanced figure of the Earth. Dependence Q_R(j) is shown in Fig.2 (that coincides with the curve divQ_R(j)). This dependence describes figure opposite of geodesic one. Potential

(11)

coincides with the sign of geodesic cardioid (Fig.2).

Thus, action of such force completely explains cardioidality of the geoid.

Arising from the action of Q_R movement upwards at middle latitudes of the northern hemisphere and downwards at middle latitudes of the southern ones owing to conservation of the momentum leads to effect of torsion: middle latitudes of the northern hemisphere turn to the west relatively of the southern one. Hence the hypothesis of equatorial shift and S-similar deformation of the meridional structure belts (Ketterfeld 1962) might be explained.

Now proceed to consider action of force of causality in the atmosphere. It is known (Khromov et al. 1971) that northern hemisphere is warmer than the southern and the thermal equator is shifted to the north relatively geographical one. The fact of heat asymmetry of the hemispheres at present has no satisfactory explanation.

However from viewpoint of causal mechanics the phenomenon of heat asymmetry is obvious. Indeed rotor of force in the atmosphere

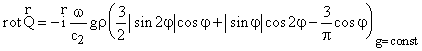(12)

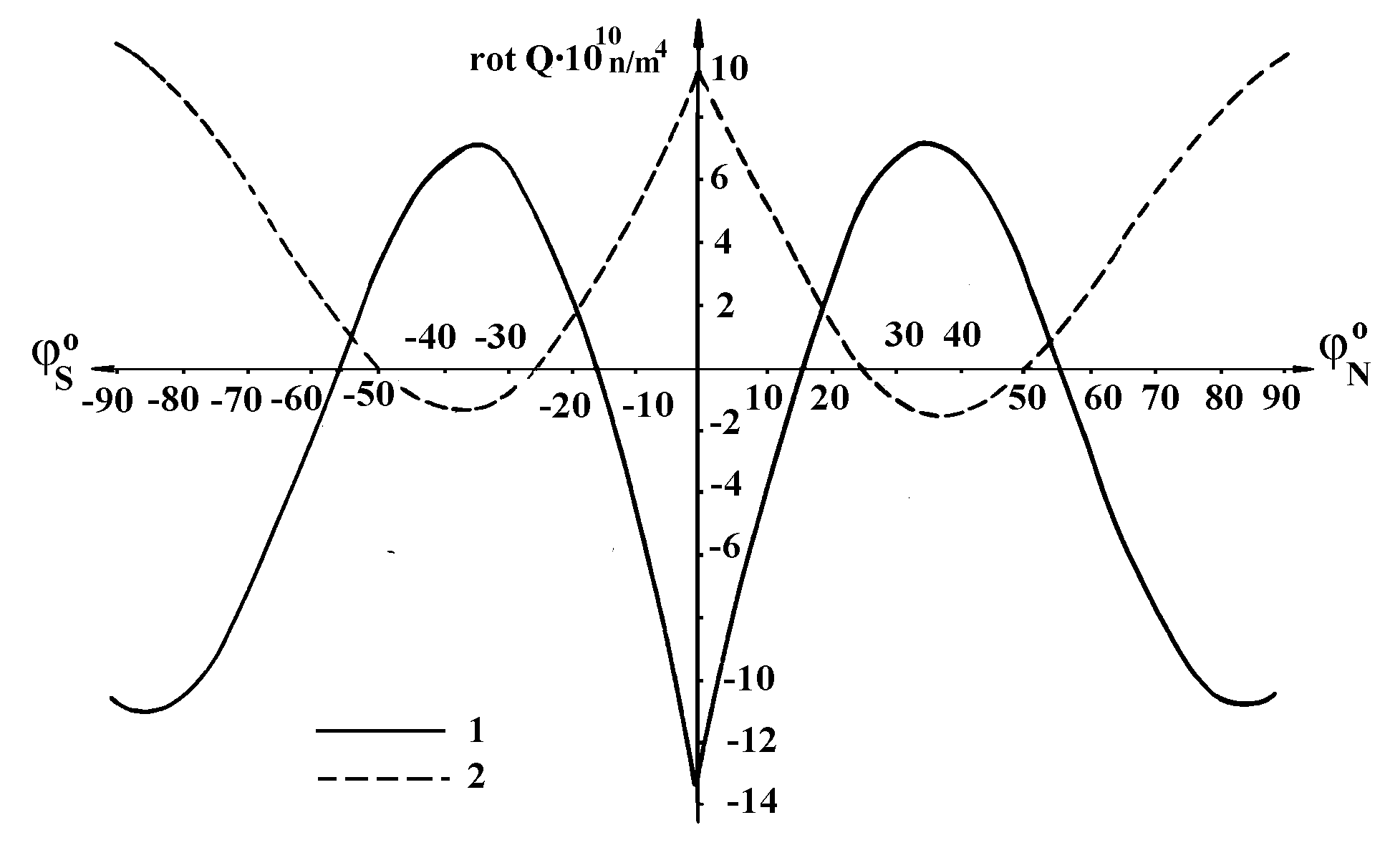
Fig.3. Zonal distribution of rotor of force of causality in the lithosphere (1, g~r) and the atmosphere (2, g=const) (for r=1kg/m³).

determines intensity of the meridional circulation. From the curve (Fig.3) it is evident that in the atmosphere as a whole positive values are prevailed. That means an existence of the integral transport from the southern hemisphere to the northern one at the lower layers of the atmosphere and opposite one at the upper layers. As a result average temperature near the Earth’s surface must be higher in the north hemisphere than in the southern one.

A visual confirmation of the above, perhaps, is southern drift of the upper frontal cloudless over the arid areas revealed by number of the satellite pictures (Morozova 1974).

In addition from the viewpoint of the considered force it may be explained that there is an existence of the intertropic convergence zone shifted to the northern hemisphere. From Fig.2 one can see that divQ_j has the break at the equator and is negative for the atmosphere into zone from 0° to 24° N.

Perhaps such distribution of divQ_j may be explained as an existence so some shift the intertropic convergence zone relatively of the equator.

Thus causal mechanics, obviously gives the possibility to explain number of the principal facts in sphere of geology and meteorology.

In addition, from our viewpoint, it is important to put into operation force of causality in dynamics of other geospheres, first of all, Earth’s core, where it may play essential role in generation of geomagnetic field. Indeed, violating symmetry, only force of causality can by natural violate the ban on generation of the field by any axial-symmetrical movements imposed by Cowling’s theorem.

REFERENCES