Research program: physical ideas, basic results, and the line of further investigations
Research is carried out, mainly, on the problem of electron and on the problem of time.
The key problem of quantum electrodynamics (QED) is the problem of electron, which can be
formulated as follows: to construct from the first principles a
non-contradictory model of electron, which takes into account experimental
facts, i.e. to find the dynamical equation capable of describing the unique
physical properties of electron, its internal structure, and its behaviour when
it interacts with electromagnetic field.
The role played by electron in the physical picture
of the world is expressed best of all by W.Thomson in the following words:
“Tell me what the electron is and I shall explain to you everything else”. It
should be emphasized that though electron became the first elementary particle
discovered experimentally (J. J. Thomson, 1897), the description of its unique
properties on the basis of a consistent quantum model remains the most
important scientific problem.
The conventional formulation of quantum electrodynamics (QED) proceeds from the
assumption that electron is a structureless point particle which does not
experience self-action. This assumption results in a serious difficulty - the
divergence of the self-energy of electron. One more difficulty of the conventional approach is
that quantum mechanics is unable to explain stability of the point-like
electron. Really, the wave packets, which could have a claim on the role of the
wave functions describing the behaviour of a free point-like electron, spread
out in time, which contradicts the experimental fact of stability of the
particle.
The difficulties mentioned above are very serious. According to Dirac, the
difficulties of QED " in view of their fundamental character can be eliminated only by the radical change of the
foundations of theory, probably, radical to the same extent as transition from
the Bohr orbits theory to modern quantum mechanics" ([1], p.403). "Correct
conclusion is," Dirac emphasizes, "that the basic equations are
erroneous. They should be changed in such a way that divergences do not appear
at all”.
As an analysis of the problem shows, one should abandon all attempts at using the
notion of point-like electron and should take into account that the
self-action of electron is the key to constructing the consistent quantum model
of the particle.
One of the boldest ideas concerning the physical nature of electron belongs to
E.Schrodinger who suggested the historically first physical interpretation of
quantum mechanics. According to the Schrodinger hypothesis, the quantity
is the density of spatial distribution of the electron’s charge ( e and
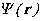are
the charge and the wave function of electron, respectively) and thus the
dimensions of electron are the same as those of atom [2,3]. However, the
attempts to substantiate this interpretation failed and for this reason Schrodinger’s
idea was rejected by the majority of physicists [4].The interest in Schrodinger’s interpretation has been revived during
the last few decades in connection with the new approaches to calculation of
radiative corrections [5-7]. A. Barut and his collaborators formulated and
developed the quantum theory of electromagnetic processes based entirely on the
self-energy picture (the Self-Field QED) [6,7]. As is pointed out by Barut [5],
“the correct quantum equation of motion for the radiating electron is not the
Dirac or the Schrodinger equation for a bare electron, but an equation containing
an additional non-linear self-energy term”.
New lines of approach to the problem of electron are proposed in [8-13]. The approach represents a synthesis of conventional quantum
electrodynamics and the ideas of the theory of self-organization in physical
systems [14].
The physical mechanism of self-organization consists in the back action
of the own field created by charged particle uponthe same particle.It
is described by the model of open system with the wave function belonging
to indefinite metric space.
The essence of the approach developed is that the
own field of electron is considered as a physical property intrinsically
inherent in the particle and, when formulating the theory, the own field is
included in the definition of electron from the very beginning. This means that
we adopt s zero approximation not a “bare” electron, but an electron capable to create the own
field and to “feel” its back influence.
Mathematically, taking into account the back action of the own field created by particle upon the same particle
results in non-linearity of the dynamical equation describing the behaviour of
electron. Thus, electron becomes a self-organizing systemwhose
physical properties, geometrical shape, and linear dimensions may be determined in a self-consistent way from
solutions of the basic dynamical equation. Electron is a quantum (elementary
excitation) of the field of charged matter localized in a bounded region of
space and subject to the Coulomb self-action.
Since electron represents a clot of electrically charged matter, creating the
long-range Coulomb forces in surroundings, its environment turns into a medium,
which can have a determining influence on physical properties of the particle.
In view of the long-range character of the Coulomb field, electron becomes
an open system inseparably bound with the environment. In a sense the whole universe takes
part in the formation of electron as a physical system.
The own field responsible for transformation of electron to an open self-organizing physical system can be
imagined as a web of electric lines of force emerging from the particle and of
magnetic ones begirding the particle. All the bodies in the Universe are
shrouded in the web and as a result the surrounding space and time as well are
endowed with physical properties.
Obviously, to describe electron as an open system one should introduce into quantum mechanics a
radically new point, namely: one should replace the model of isolated system,
which is at the heart of modern physics and described by harmonic oscillator,
with the model of open system. It is pertinent to note here that the theory of
quantum particles based on the use of the models of isolated system is,
strictly speaking, physically meaningless. Really, any observation conducted on
a system represents a process of interaction of the system with the means of
observation. In the case of microparticles (quantum particles) this interaction
is not weak and consequently it is inadmissible to neglect it, i.e.
microparticles should be necessarily considered as open systems [15].
As open system has the richer physical content in comparison with isolated system, the essentially new
mathematical ideas are needed for such a system to be described. To take into
account that real electron, being considered as an open system, is inseparably
linked with surrounding medium, we should first of all increase the number of
dynamical variables describing it. Indeed, real electron can be imagined as a
system consisting of two components: one of them should correspond, in a sense,
to the particle alone (to the “bare” particle) and the other to the surrounding
medium, in which the particle moves. Hence, it is necessary to introduce the
dynamical variables relating to the electron itself and the dynamical variables
relating to environment. In the papers [8-13,15,16] an approach is developed in
which the number of dynamical variables is doubled. To each dynamical variable
of the “bare” particle, 
,there correspond two
dynamical variables, and 
, of which refers to the particle itself and
to the environment, with
and being interpreted as components of the
wave function describing the quantum state of particle.
Besides, the system under study should be subordinated to a condition for openness
expressing thefact that real electron is indissolubly bound to environment and its
interaction with environment cannot be weak. The condition for openness can be
formulated as follows: open system should make sense only in the event that
there are simultaneously both components – the particle alone and the
environment, and these components should be equivalent.
In the approach developed the fulfillment of the condition for openness
is provided with that the wave function space with positively defined
quadratic form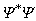 
is replaced by the space
with indefinite metrics
 |
(1) |
In [9,11-13], from the action principle, the basic dynamical equation is derived taking into account
the relativity principle and describing the self-acting electron as an open
self-organizing system. This equation is of the form (only for the component
) :
 |
(2) |
where |
 |
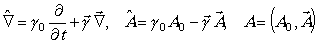is the 4-vector, |
 |
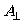and |
 |
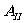are the vortex 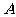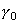and |
, 
and 
are Dirac’s matrices, 
is the electromagnetic field tensor,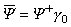 
. By its appearance
this equation coincides with the usual Dirac equation for a charged particle in an
external field described by 4-potential. However, in reality, it differs essentially from
Dirac’s equation. The distinction consists in that the equation derived is non-linear and
non-local, with the non-locality being of spatial and temporal character.It should be noted that the potential and vortex components of the 4-potential
entering into the dynamical equation differ from each other by their physical
nature: the first describes the own field and is expressed in terms of the wave
function components of electron, and the second describes the vortex electromagnetic
field and is uniquely determined by the electromagnetic field variables. From the
formal point of view, the content of the QED formulation developed here is that
these two essentially different quantities are integrated into a single 4-vector.
As a detailed analysis shows, solutions to the basic dynamical equation of electron
describe the clots of self-acting electrically charged matter, localized in space,
i.e. electron is a soliton [16]. The self-acting electron can be in different
quantum states characterized by internal energy, dimensions, and geometric shape.
The internal energy spectrum of electron is discrete with an infinitely large number
of levels. To each value of internal energy there correspond certain linear dimensions
and geometric shape of the region of localization of electron’s charge. Dimensions and
the number of extrema of wave function increase with increasing the value of internal
energy [8,16].
The distribution of electric charge of electron in the ground state consists of the
range of basic localization with the linear dimensions of the order of Bohr radius
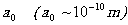and of the tail stretching up to infinity.
Owing to the non-linearity of the dynamical equation of electron, the wave
function does not obey the superposition principle. In virtue of this electron
acquires the properties of absolutely rigid body: the perturbation acting on
electron at an instant 
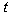in the range of
basic localization becomes known at the next instant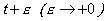 
at any distance from it.
